KOREA CAPITAL MARKET INSTITUTE

Problems in leveraged and inverse products

At the height of the Covid-19 pandemic, leveraged and inverse exchange-traded funds (ETFs) are gaining popularity among retail investors. For example, KODEX F-200 Inverse 2X, one of the typical leveraged inverse products in Korea, saw its net asset value almost triple from KRW 0.7 trillion at the end of 2019 to KRW 2.3 trillion at the end of May 2021. By contrast to the economic slowdown due to Covid-19, stock markets continued to rally, which might have nudged retail investors to feel ironic and bet on inverse. Thanks to them, the overall NAV of leveraged and inverse ETFs jumped 30% to KRW 8.9 trillion from KRW 6.8 trillion during the same period, with the daily average trading value almost tripling from KRW 0.7 trillion in 2019 to KRW 2.9 trillion in 2020. 
Figure 1. NAV of leveraged and inverse ETFs
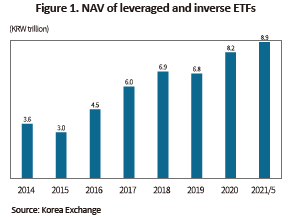
  However, the steep growth observed in leveraged and inverse products is not all welcoming. All of the leveraged and inverse products listed in Korea have two serious adverse effects in common. First, the innate compounding effect in those products have an adverse impact on investors’ long-term performance. Second, the abrupt growth in the NAV also elevated the size of rebalancing transactions, which would increase the risk of stock market volatility. As details on this issue were already discussed in Kwon (2020a; 2020b), this article places its focus on a fix to the problem.


A proposed improvement for curtailing excessive rebalancing

The fundamental cause behind the adverse effects of leveraged and inverse products lies in frequent rebalancing transactions that are executed daily by those products with an aim to maintain the multiple, which takes a similar shape to trend following. For example, if an underlying index rises 1%, a 2x leveraged inverse¹⁾ product would have to buy an addition of 6% of the NAV. On the other hand, it would have to sell 6% of the NAV when there is a 1% fall in the underlying index.²⁾ The size of rebalancing tends to rise in proportion to changes in the underlying index. Assume that a stock market fluctuates to lose 5% one day and then rebounds to the original level on the next trading day. Then the leveraged inverse product would have to execute rebalancing equivalent to 65% of the initial NAV. If it is possible to improve how leveraged and inverse products are rebalanced, and thus reduce the now excessive size of rebalancing, this will help relieve a significant part of the adverse effects mentioned above.

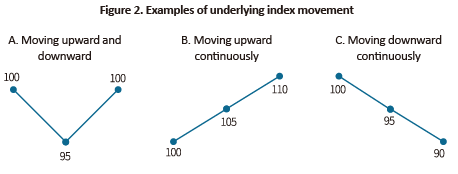
One simple fix to the problem could be to reduce the size of excessive rebalancing: An extension of the rebalancing cycle from one day to a longer period is worth considering. As illustrated in Figure 2-A, when an underlying index falls and then rises again, a leveraged inverse product executes daily rebalancing twice from 100 to 95, and then from 95 to 100, where the size of rebalancing per trading day reaches about 30% of the NAV. However, assume that the product extends the rebalancing cycle to two days. It would not rebalance on the first trading day when the index fell from 100 to 95, while rebalancing takes place on the second trading day when the index rebounds from 95 to 100. For the two-day cycle, the index moves from 100 to 100 so that the product doesn’t have to rebalance at all. This creates an effect of reducing unnecessary rebalancing.
 
However, an extension of the rebalancing cycle alone cannot resolve all issues. This may exacerbate the adverse effects in other cases, for example, when an underlying index moves in the same direction for two days as shown in Figure 2-B and C. If rebalancing is executed every other day, the product would have to deal with even more drastic changes—from 100 to 110, or from 100 to 90—on one trading day, which increases the size of rebalancing to 60% of the NAV on the second trading day. This may give rise to the risk of amplifying volatility in underlying indexes.
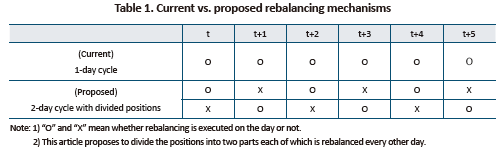
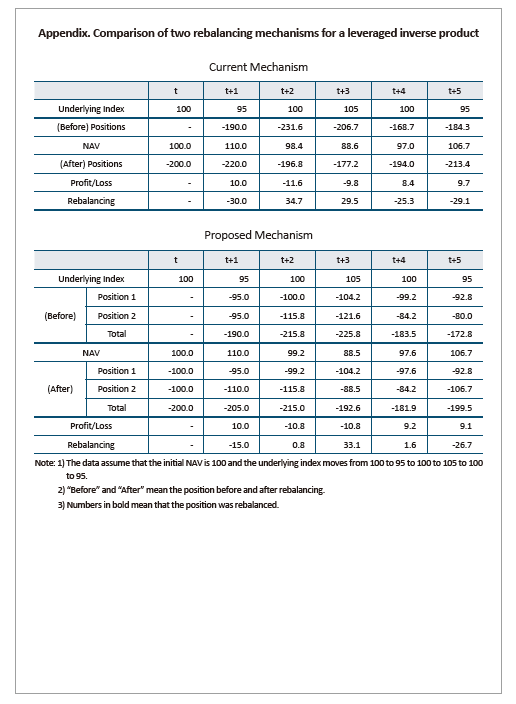
  Aiming to overcome such a shortcoming, this article proposes a new mechanism that divides trade positions on top of the extended rebalancing cycle. For example, the rebalancing cycle is increased to two days and the positions are divided into two parts each of which is to be rebalanced every other day. As shown in Table 1, one half of the positions is rebalanced on T, T+2, and T+4, while the other half is rebalanced on T+1, T+3, and T+5. This effectively reduces the size of unnecessary rebalancing if an underlying index moves up and down repeatedly as shown in Figure 2-A. If the index keeps rising or falling as seen in Figure 2-B and C, the proposed mechanism can fix the problem where rebalancing for two trading days is concentrated on one trading day.³⁾ It is possible to change the rebalancing cycle to three days: In that case, the positions are cut into three parts to be rebalanced as mentioned above. Likewise, the cycle should be extended to four or five days, or even longer.
  Backtesting results

To see how the new rebalancing mechanism reduces the adverse effects of leveraged and inverse products, this article executed backtesting.⁴⁾ The analysis used KOSPI 200 Futures, and the period of backtesting spans over a total of 3,561 trading days between January 2007 and May 2021. Although this article includes the results of a leveraged inverse product for convenience, the findings described here remained similar across other leveraged and inverse products. The rebalancing cycle here is fixed to two days.
 
First, it is worth looking at the absolute size of reduction in rebalancing. If an investor buys a leveraged inverse product worth of KRW 1 million on a trading day during the analysis period, the size of rebalancing during the next 30 trading days stood at KRW 1.68 million under the current mechanism and KRW 1.20 million under the proposed mechanism. This means that the proposed mechanism achieved a 30% reduction in rebalancing trades. In particular, the reduction in unnecessary rebalancing was more substantial when the underlying index went up and down alternately on a daily basis.

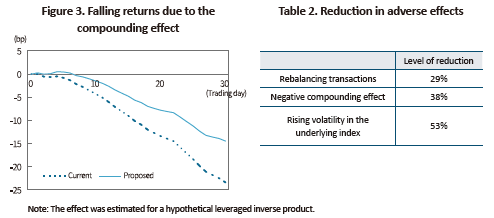
Then, did the reduction in rebalancing lead to a corresponding decrease in the adverse effects of leveraged and inverse products? To answer the question, it is needed to look at how investment returns fell due to the compounding effect. Figure 3 indicates the average compounding effect of a leveraged inverse product bought on a certain day. For 30 trading days after the purchase, the size of compounding effect was –23 bp under the current mechanism and and –14 bp under the proposed one, which means a 40% reduction in the negative effect. If annualized, this translates into a 75 bp increase in investment returns. However, that improvement reflects the compounding effect only. If other unexpected effects derived from decreased rebalancing—such as reduced transaction costs and less burden on labor costs—were fully considered, the actual size of improvement in investment performance should be much larger than that.
 
In another analysis, the impact of leveraged and inverse products on the volatility of their underlying indexes was explored. If a high (low) return on an underlying index leads to a positive (negative) rebalancing transaction of a product, this could evidence the product’s contribution to increasing volatility of the underlying index. To explore the impact, this article measures and compares the covariance between daily returns on the underlying index, and the ratio of rebalancing positions to the previous day’s NAV. During the analysis period, the covariance stood at 0.00113 under the current mechanism, and 0.00053 under the proposed one. This means that the new mechanism, compared to the current one, halved the risk that rebalancing raises underlying index volatility.⁵⁾
  Conclusion

This article confirmed that the new mechanism proposed here effectively reins in unnecessary rebalancing of leveraged and inverse products, and thus improves the side effects to a substantial extent. The proposed mechanism, if adopted in practice, is projected to curtail the reduction in investors’ long-term return to a substantial extent, and to impose a positive impact on financial market stability going forward.
 
However, the new mechanism will not be able to be adopted by a real product unless the government chooses to relax relevant regulation. The current regulation limits the total exposure of derivatives in ETFs up to 200%. If the rebalancing cycle under the new mechanism is extended to more than two days, the exposure of some products whose multiple is equal to ±2 will temporarily exceed 200%. Under the new mechanism, however, in most cases the exposure can be managed about the 200% level and even if the exposure temporarily rises above the threshold, it gets back to the normal level on the next trading day.⁶⁾ In that sense, deregulation in this area would not be a far-fetched claim.
 

---

1) “Leveraged inverse” ETFs refer to a product seeking to the magnified opposite return of an underlying index. Because a 2x (two times) leveraged inverse ETF is most popular in Korea, this product is called a leveraged inverse ETF in this article for convenience.
2) When the NAV of the leveraged inverse product is 100, its exposure to the underlying asset has to be -200. If the underlying asset rises 1% in value on the next trading day, the exposure will turn to -202 while the NAV will decrease to 98. If no action is taken, the multiple will deviate to –2.06 (-202/98). Hence, to maintain the stated multiple (-2x), the exposure should be lowered to –196 (-2×98), which can be done by a rebalancing transaction (+6). By the same token, a 1% decline requires a rebalancing transaction (-6) for maintaining the stated multiple (-2x).
3) More details on the proposed mechanism can be seen in a case in Appendix attached at the end of this article. A detailed look at the case confirms that the size of rebalancing under the proposed mechanism decreased significantly on T+2 and T+4 when the underlying index moved up and down, but remained unchanged on T+3 and T+5 when the index moved in the same direction. 
4) Derived from the question of what if a new method was applied in the past, this methodology is often used to verify a new method. 
5) The 50% reduction in the risk of higher volatility was even higher than the 30% reduction in rebalancing shown earlier, which is quite intriguing. This means the proposed mechanism serves to not only rein in unnecessary rebalancing, but also to a larger extent reduce the risk of rising volatility in the underlying index. This result is because under the proposed mechanism, unlike the current one, rebalancing positions are not exactly in proportion to changes in the underlying index.
6) When the new mechanism was applied to a leveraged inverse product during the analysis period, the multiple stood at -2.00 on average, with the standard distribution at 0.04. Over 97% of the trading days have the multiple hovering between -2.1 and -1.9.


References

M.K., Kwon, 2020a, Impact of Rebalancing on Leveraged and Inverse Funds, KCMI Capital Market Focus 2020-12.
M.K., Kwon, 2020b, Leveraged and inverse ETP: Current state and risk factors, KCMI Issue Paper 20-17.